Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Generate the hat tiling using combinatorial hexagons
ResourceFunction["HatHexagons"][ind] plots the hexagonal initial condition for integer ind between 1 and 10. | |
ResourceFunction["HatHexagons"][ind, depth] plots the resulting configuration of hexagons after applying the inflation rule an integer number of times, depth ≥ 0. | |
ResourceFunction["HatHexagons"][ind, depth, rotation] rotates the resulting configuration by an angle rotation × π/3. | |
ResourceFunction["HatHexagons"][ind,…, type] changes the view to show "Hat" or "Cluster" tiles instead of hexagons. |
Plot one of ten initial conditions as a hexagon:
| In[1]:= |
| Out[1]= | 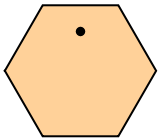 |
Compare different views of the same combinatorial hexagon:
| In[2]:= |
| Out[2]= | 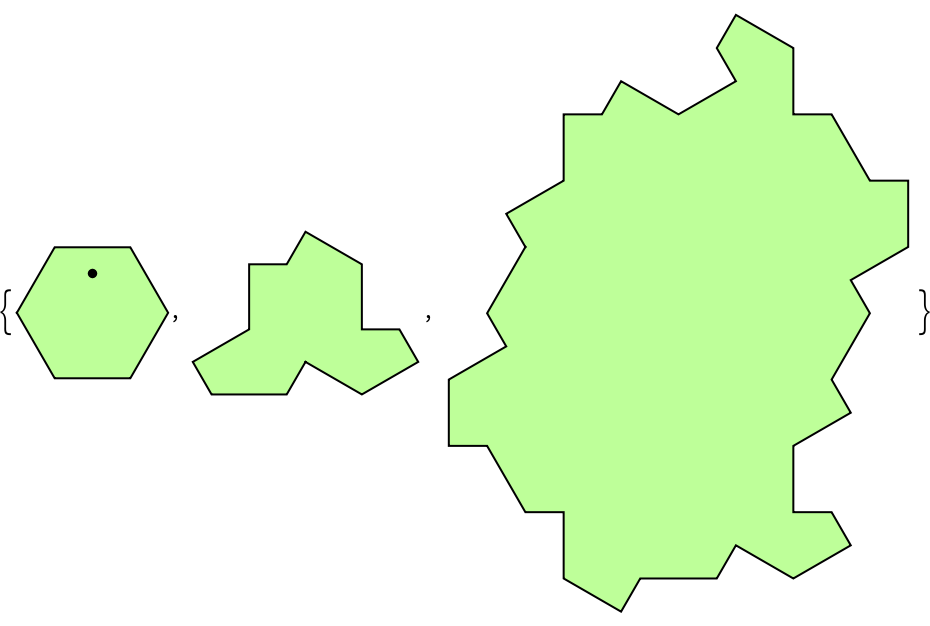 |
Obtain successively larger fragments of a hat-hexagon tiling:
| In[3]:= |
| Out[3]= | 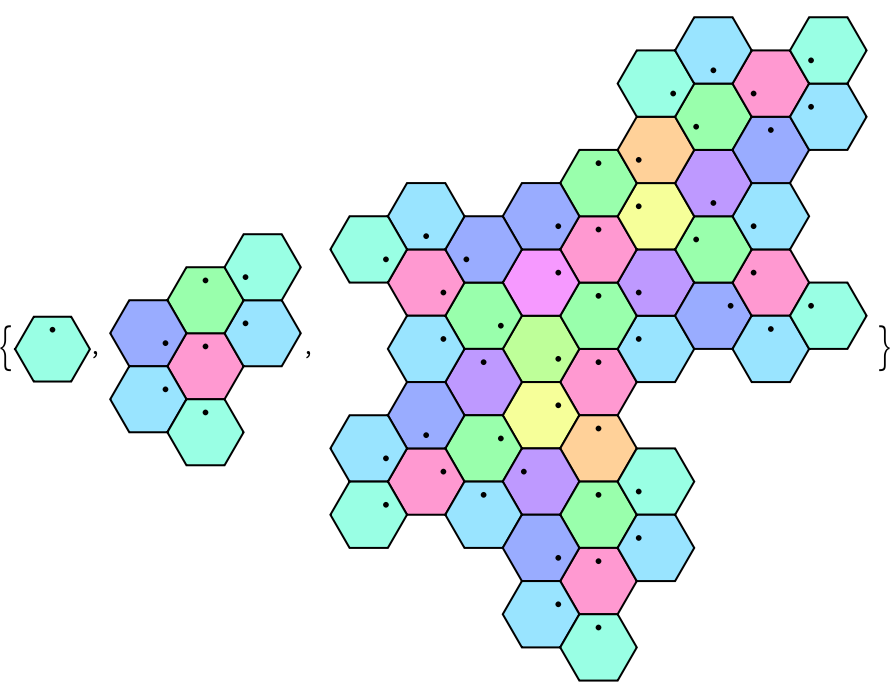 |
Compare with hat view:
| In[4]:= |
| Out[4]= | 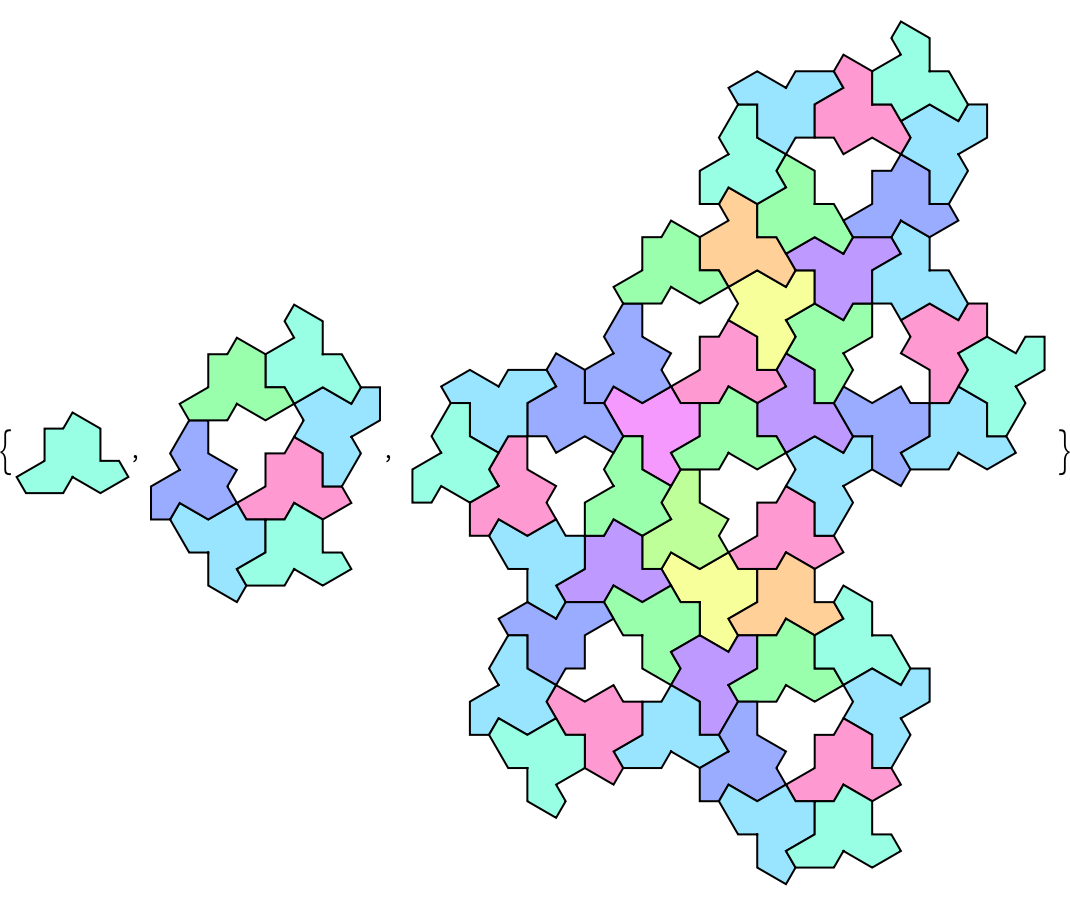 |
And compare with cluster view:
| In[5]:= |
| Out[5]= | 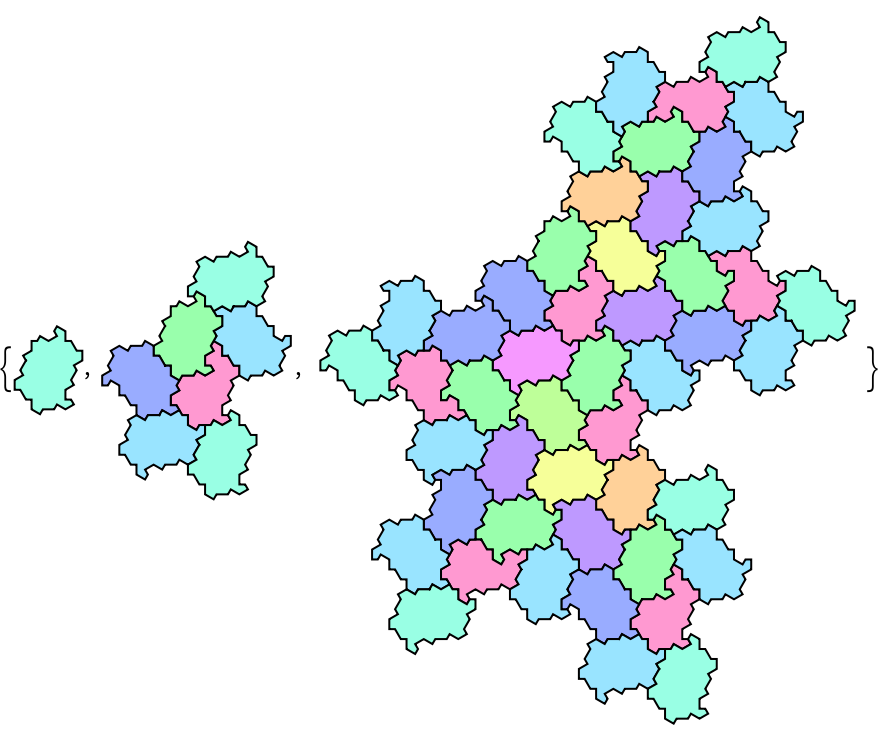 |
Plot the ten initial conditions:
| In[6]:= |
| Out[6]= | 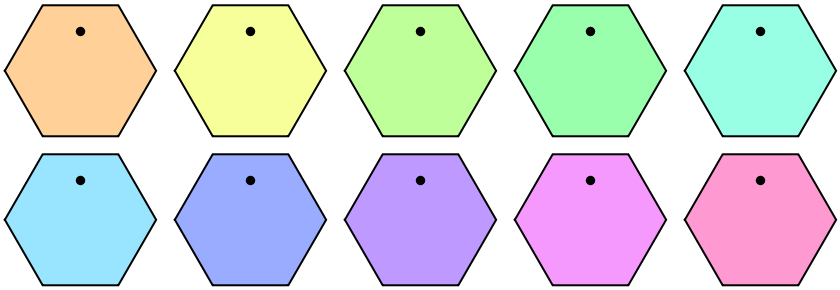 |
Their inflation images are not entirely unique:
| In[7]:= |
| Out[7]= |  |
Each tile can be placed in six distinct orientations:
| In[8]:= |
| Out[8]= | 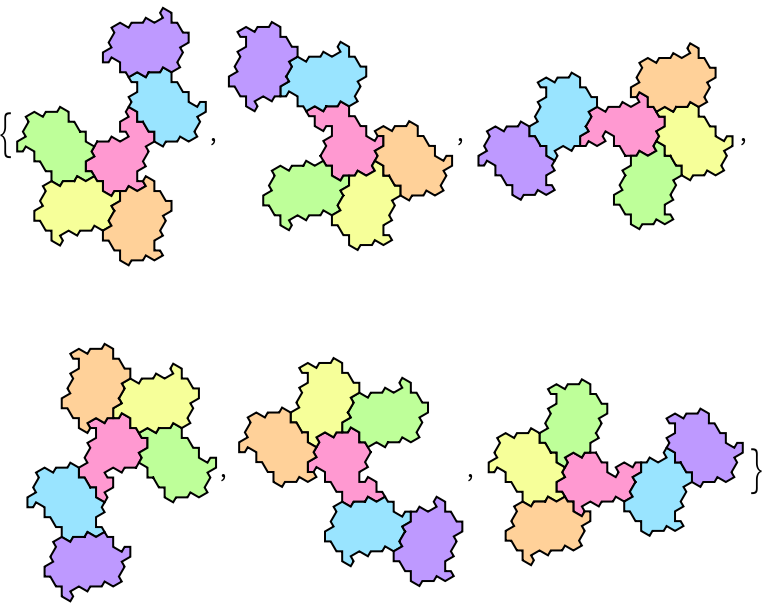 |
Color the hat tiling using GrayLevel:
| In[9]:= | ![ResourceFunction["HatHexagons"][1, 2, 2, "Hat", ColorRules -> (
x_ :> GrayLevel[x/12]
), ImageSize -> 500]](https://www.wolframcloud.com/obj/resourcesystem/images/418/41867d37-2300-4b56-a463-a1dd6cb1f0dd/77dcfa6edafb9a19.png) |
| Out[9]= | 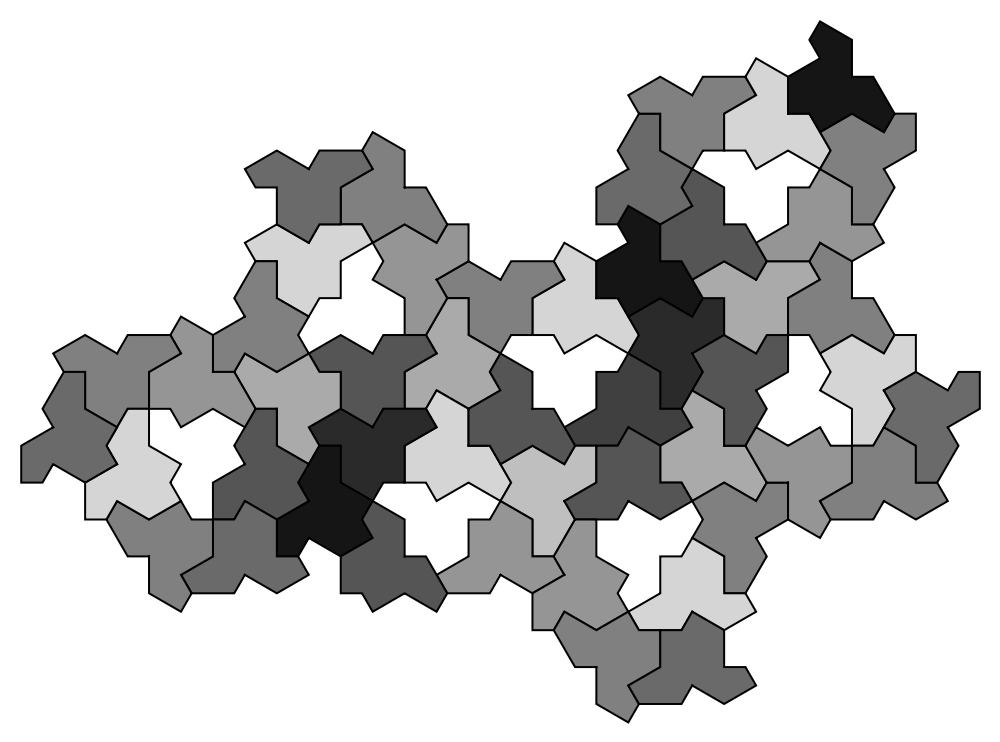 |
Add red EdgeForm:
| In[10]:= | ![ResourceFunction["HatHexagons"][1, 2, 2, "Hat", ColorRules -> (
x_ :> {EdgeForm[Darker[Red]], GrayLevel[x/12]}
), ImageSize -> 500]](https://www.wolframcloud.com/obj/resourcesystem/images/418/41867d37-2300-4b56-a463-a1dd6cb1f0dd/2d8bdb9a68816826.png) |
| Out[10]= | 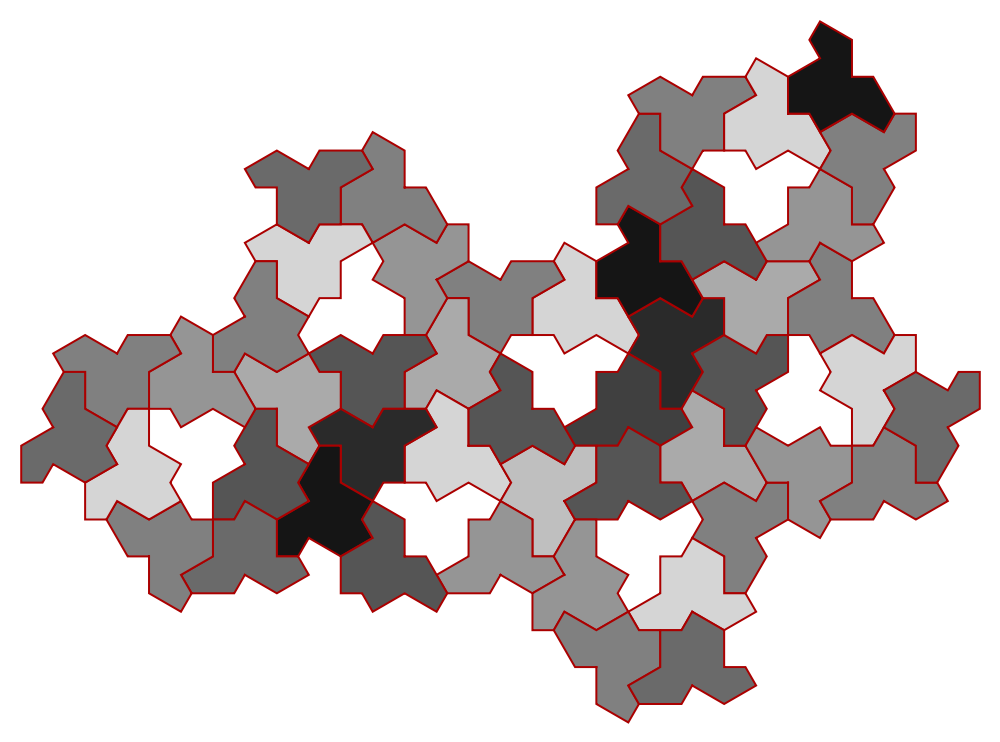 |
The same style can also be introduced using ColorFunction:
| In[11]:= | ![ResourceFunction["HatHexagons"][1, 2, 2, "Hat", ColorFunction -> Function[
{EdgeForm[Darker[Red]], GrayLevel[#/12]}],
ImageSize -> 500]](https://www.wolframcloud.com/obj/resourcesystem/images/418/41867d37-2300-4b56-a463-a1dd6cb1f0dd/625a5df30b8c07c3.png) |
| Out[11]= | 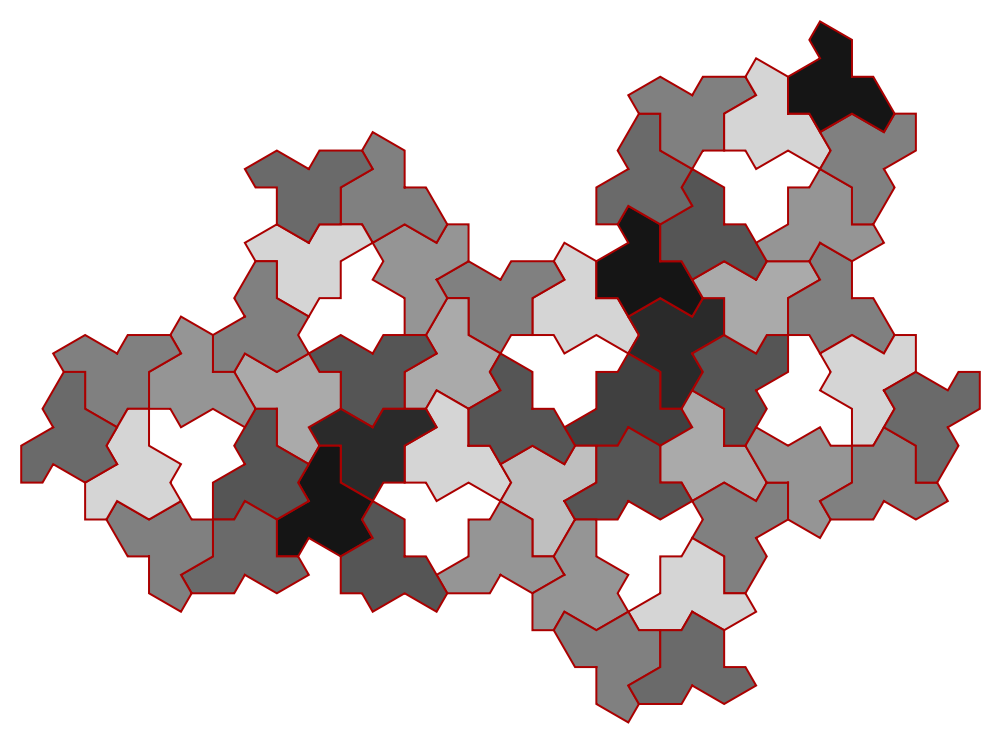 |
Only three views are available:
| In[12]:= |
| Out[12]= |
Show a hexagon substitution:
| In[13]:= | ![With[{ind = 6},
ResourceFunction["HatHexagons"][1, 4, 2, ColorFunction -> Function[
If[SameQ[#, ind],
{EdgeForm[Gray], Gray},
{EdgeForm[Gray], White}]],
ImageSize -> 600]]](https://www.wolframcloud.com/obj/resourcesystem/images/418/41867d37-2300-4b56-a463-a1dd6cb1f0dd/07a0389262ecba14.png) |
| Out[13]= | 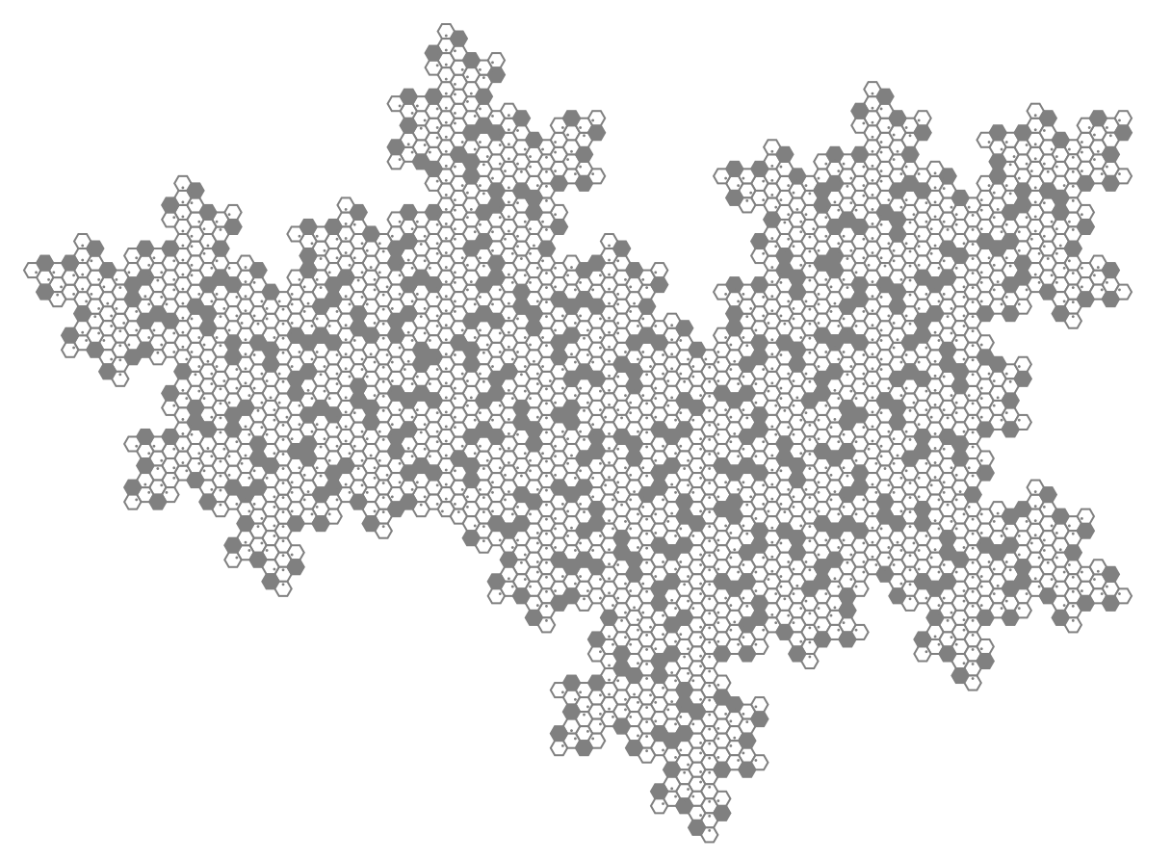 |
This work is licensed under a Creative Commons Attribution 4.0 International License