Basic Examples (2)
Obtain a formula for the Frobenius solution for the ODE 2x2y''+7x(x+1)y'-3y=0 near the singular point x=0:
The formula describes a Frobenius series 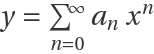 . Display the same formula in a form compatible with the resource function FrobeniusDSolve (see Properties and Relations):
. Display the same formula in a form compatible with the resource function FrobeniusDSolve (see Properties and Relations):
Scope (3)
The function can operate with complex numbers:
The inputs of the function can be symbolics:
FrobeniusDSolveFormula can be used to solve ODEs of any order. Here is the formula for the Frobenius series solution to (x-1)4f''''[x]=x(2+x)f[x]+x2(x-1)2f''[x]-19(x-1)3f'''[x] near the singular point x=1:
Options (1)
If the option "ComputeError" is set to True, FrobeniusDSolveFormula will not only output a formula for the Frobenius series solution but also the formula for the error of this solution:
The formula doesn't make much sense to a human and instead is meant to be fed into the resource function FrobeniusDSolve, which will in turn compute an approximate solution of the form 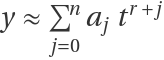 and the error of this solution.
and the error of this solution.
Applications (2)
FrobeniusDSolve is a related resource function that computes approximate Frobenius and power series solutions to ODEs. The solutions it outputs are of the form 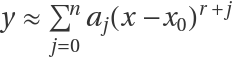 for some parameters x0, j. The following is an example of how FrobeniusDSolve can be used to obtain approximate solutions to the ODE x2y''-2xy'+(1+x)y=0 with x0=0 and n=4:
for some parameters x0, j. The following is an example of how FrobeniusDSolve can be used to obtain approximate solutions to the ODE x2y''-2xy'+(1+x)y=0 with x0=0 and n=4:
When solving an ODE, FrobeniusDSolve first utilizes FrobeniusDSolveFormula to compute a formula for the solution. The formula is then used to calculate the approximate solutions. When a single ODE is to be solved many times with different parameters, computation time can be significantly reduced by computing the formula separately, using FrobeniusDSolveFormula. This way, FrobeniusDSolve doesn't have to compute the formula every time, and it only has to do the numerical part of the computation. As can be seen from the following example, the decrease in computation time is dramatic:
Properties and Relations (2)
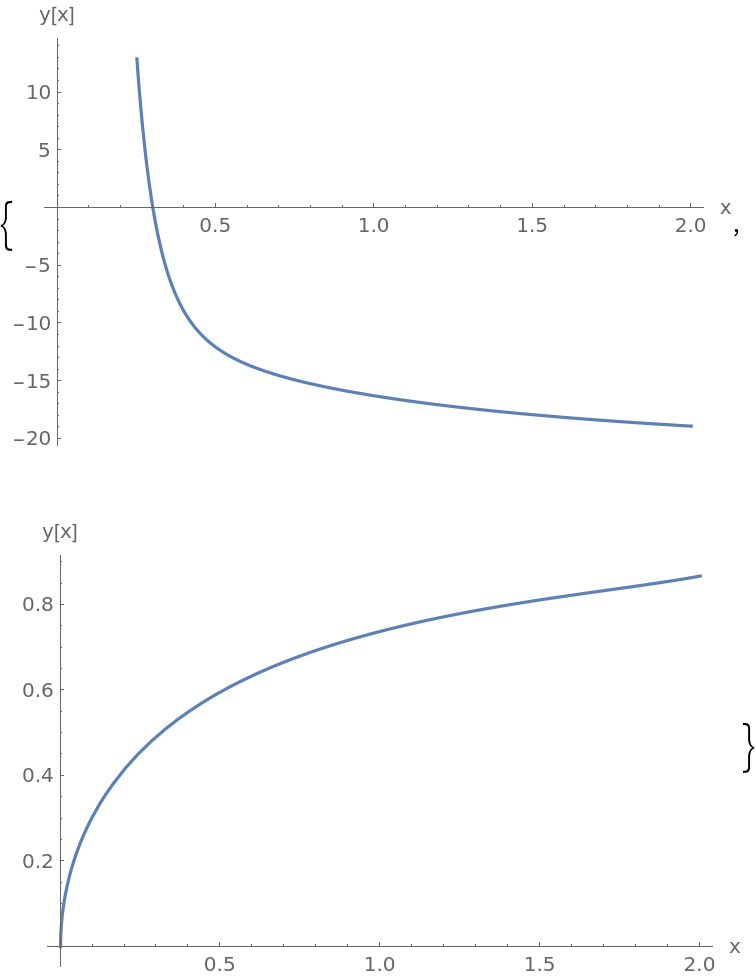
Formulas given by FrobeniusDSolveFormula can be fed into the resource function FrobeniusDSolve to compute approximate Frobenius series solutions. This can help significantly improve efficiency when a single ODE is to be solved repeatedly (see Applications). Obtain a formula for the Frobenius solution for the ODE 2x2y''+7x(x+1)y'-3y=0 near the singular point x=0, and output it in a form compatible with FrobeniusDSolve:
Use the formula to compute two linearly independent approximate solutions 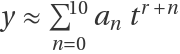 :
:
Possible Issues (2)
The ODE must be linear and homogeneous, and its coefficients must be polynomial. Otherwise, FrobeniusDSolveFormula returns $Failed:
If the ODE isn't liner but can be made so by dividing by a non-zero factor, the function still doesn't accept it:
![]() . Display the same formula in a form compatible with the resource function FrobeniusDSolve (see Properties and Relations):
. Display the same formula in a form compatible with the resource function FrobeniusDSolve (see Properties and Relations):
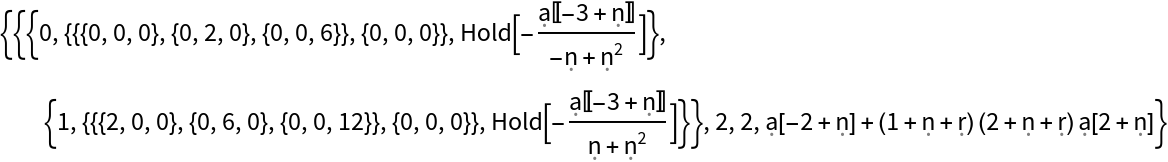
![]() and the error of this solution.
and the error of this solution.![]() for some parameters x0, j. The following is an example of how FrobeniusDSolve can be used to obtain approximate solutions to the ODE x2y''-2xy'+(1+x)y=0 with x0=0 and n=4:
for some parameters x0, j. The following is an example of how FrobeniusDSolve can be used to obtain approximate solutions to the ODE x2y''-2xy'+(1+x)y=0 with x0=0 and n=4: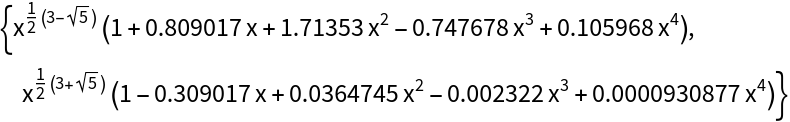
![Block[{eqn = (y''[x] + (1 + x^2) y[x] == 0), ncoefs = 100, formula},
formula = ResourceFunction["FrobeniusDSolveFormula"][eqn, y, x, 0];
{1000 First@RepeatedTiming[FrobeniusDSolve[x, 0, ncoefs, formula]],
1000 First@RepeatedTiming[FrobeniusDSolve[eqn, y, x, 0, ncoefs]]}
]](https://www.wolframcloud.com/obj/resourcesystem/images/631/631a2270-e678-49ed-9e58-417abdb78a14/45a7f68a491a37cc.png)
![]() :
: