Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Evaluate Bulirsch's general incomplete elliptic integral
ResourceFunction["BulirschEL"][x,m,p,a,b] gives Bulirsch's general incomplete elliptic integral |
Evaluate numerically:
| In[1]:= |
|
| Out[1]= |
|
| In[2]:= |
|
| Out[2]= |
|
Evaluate numerically for complex arguments:
| In[3]:= |
|
| Out[3]= |
|
Evaluate to high precision:
| In[4]:= |
|
| Out[4]= |
|
The precision of the output tracks the precision of the input:
| In[5]:= |
|
| Out[5]= |
|
Simple exact results are generated automatically:
| In[6]:= |
|
| Out[6]= |
|
| In[7]:= |
|
| Out[7]= |
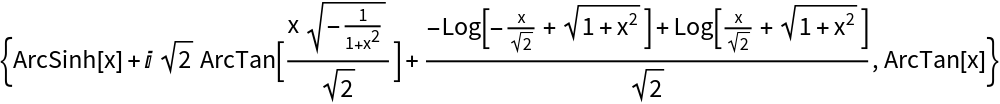
|
BulirschEL threads elementwise over lists:
| In[8]:= |
|
| Out[8]= |
|
Series expansion of BulirschEL at the origin:
| In[9]:= |
|
| Out[9]= |
|
All incomplete elliptic integrals can be expressed in terms of BulirschEL:
| In[10]:= |
|
| Out[10]= |
|
| In[11]:= |
|
| Out[11]= |
|
| In[12]:= |
|
| Out[12]= |
|
Linear combinations of incomplete elliptic integrals can be expressed in terms of BulirschEL:
| In[13]:= |
![With[{\[Phi] = \[Pi]/5, m = 2/3, a = 4, b = 5}, N[{a EllipticF[\[Phi], m] + b EllipticE[\[Phi], m], ResourceFunction["BulirschEL"][Tan[\[Phi]], 1 - m, 1, a + b, a + b (1 - m)]}]]](https://www.wolframcloud.com/obj/resourcesystem/images/ffc/ffcde3de-1764-4620-b281-a15cb778063e/239f9119ad850408.png)
|
| Out[13]= |
|
| In[14]:= |
![With[{n = 4/5, \[Phi] = \[Pi]/5, m = 2/3, a = 4, b = 5}, N[{a EllipticF[\[Phi], m] + b EllipticPi[n, \[Phi], m], ResourceFunction["BulirschEL"][Tan[\[Phi]], 1 - m, 1 - n, a + b, a (1 - n) + b]}]]](https://www.wolframcloud.com/obj/resourcesystem/images/ffc/ffcde3de-1764-4620-b281-a15cb778063e/21c4a9050390bea8.png)
|
| Out[14]= |
|
Wolfram Language 12.3 (May 2021) or above
This work is licensed under a Creative Commons Attribution 4.0 International License