Basic Examples (2) 

A symmetric definite pencil:

In[1]:=

Reduce the pencil:

In[2]:=

Out[2]=

Compute the eigenvalues of the transformed matrix:

In[3]:=

Out[3]=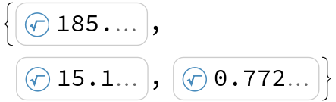

These are the same as the eigenvalues of the original pencil:

In[4]:=

Out[4]=

Compute the normalized eigenvectors of the transformed matrix:

In[5]:=

Out[5]=

These are the same as the eigenvectors of the original pencil, up to a normalization constant:

In[6]:=

Out[6]=

A machine precision symmetric definite pencil:

In[7]:=

Reduce the pencil:

In[8]:=

Out[8]=

Compute the eigenvalues of the transformed matrix:

In[9]:=

Out[9]=

These are the same as the eigenvalues of the original pencil:

In[10]:=

Out[10]=

Compute the normalized eigenvectors of the transformed matrix:

In[11]:=

Out[11]=

These are the same as the eigenvectors of the original pencil, up to a normalization constant:

In[12]:=

Out[12]=

Scope (3) 

Reduce an exact symmetric definite pencil:

In[13]:=

Out[13]=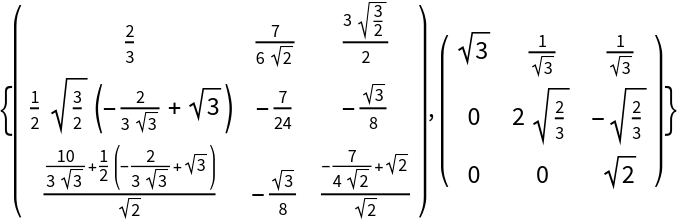

Reduce a symmetric definite pencil with 24-digit precision arithmetic::

In[14]:=

Out[14]=

A random symmetric definite pencil:

In[15]:=

Out[15]=

Reduce the pencil:

In[16]:=

Out[16]=

A random Hermitian definite pencil:

In[17]:=

Out[17]=

Reduce the pencil:

In[18]:=

Out[18]=

Options (2) 

Method (2) 

Use the "Cholesky" method to reduce the definite pencil:

In[19]:=

Out[19]=

---

Use the "Eigensystem" method to reduce the definite pencil:

In[20]:=

Out[20]=

Properties and Relations (4) 

A definite pencil:

In[21]:=

Reduce the definite pencil:

In[22]:=

Out[22]=

Verify that m is given by ConjugateTranspose[t].s.t:

In[23]:=

Out[23]=

Verify that a is given by ConjugateTranspose[t].t:

In[24]:=

Out[24]=

Possible Issues (1) 

DefinitePencilReduce is left unevaluated if the argument is not a definite pencil:

In[25]:=

Out[25]=

In[26]:=

Out[26]=