Fast numerical estimation of the power spectral density or the cross spectral density
Contributed by:
Julien Kluge
Quantum Optical Metrology; Joint Lab Integrated Quantum Sensors
Department of Physics
Humboldt-Universität zu Berlin
julien@physik.hu-berlin.de
Examples
Basic Examples (1)
Calculate the spectral density of a random data sample:
Scope (2)
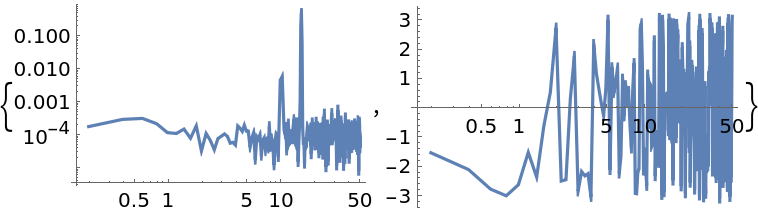
Evaluate the Cross Spectral Density (CSD) of two sinusoidal, noisy datasets and display amplitude and phase:
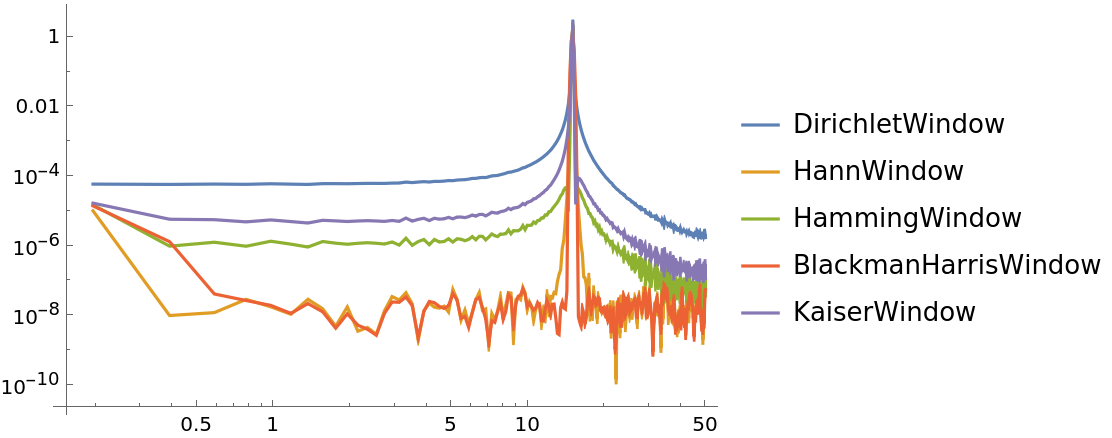
Compare the influence of different spectral windows on the PSD:
Options (12)
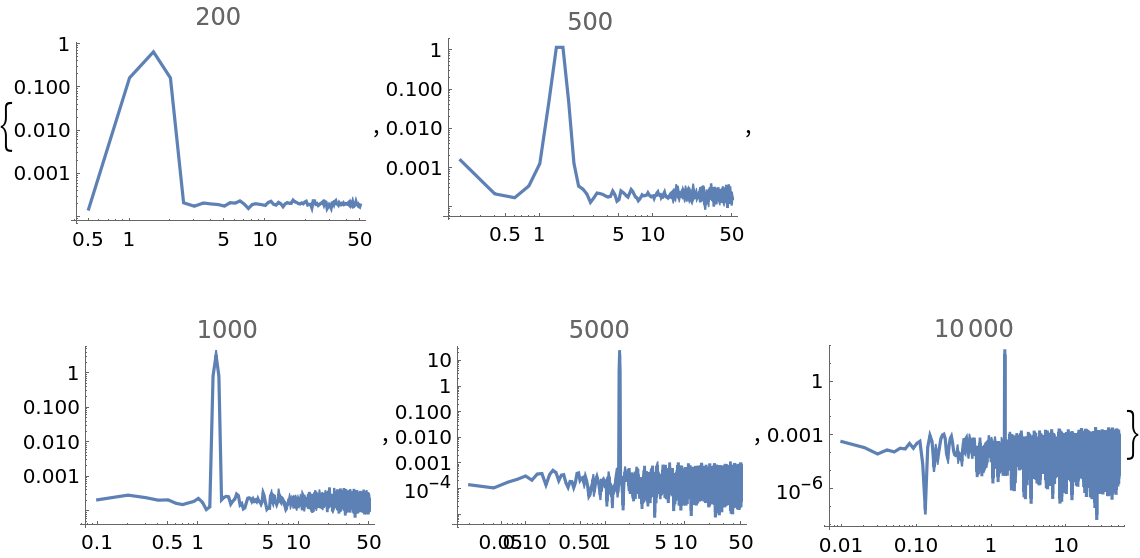
SegmentSize (1)
"SegmentSize" sets number of data points from which to calculate the Fourier transform; smaller numbers means more averaging but lower resolution:
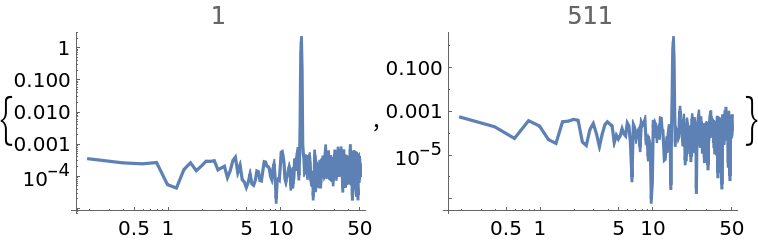
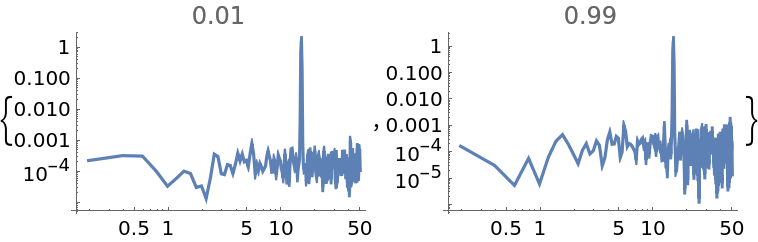
OverlapOffset (2)
"OverlapOffset" allows to set the distance of the starting point between two partitioned segments; a smaller integer number means closer overlaps and thus more averaging:
"OverlapOffset" also allows the specification of an overlap fraction of the number of data points minus the segment size, as a real number between zero and one:
Window (3)
Use the "Window" option to specify windowing functions:
A list can be applied as the window function, provided the length is equal to its segment size:
Set a custom function as the window:
OneSided (1)
Set "OneSided" to False to get a two sided evaluation:
Reduction (1)
Specify Median as a reduction function:
DensityScaling (1)
Set "DensityScaling" to False to get a spectrum scaling:
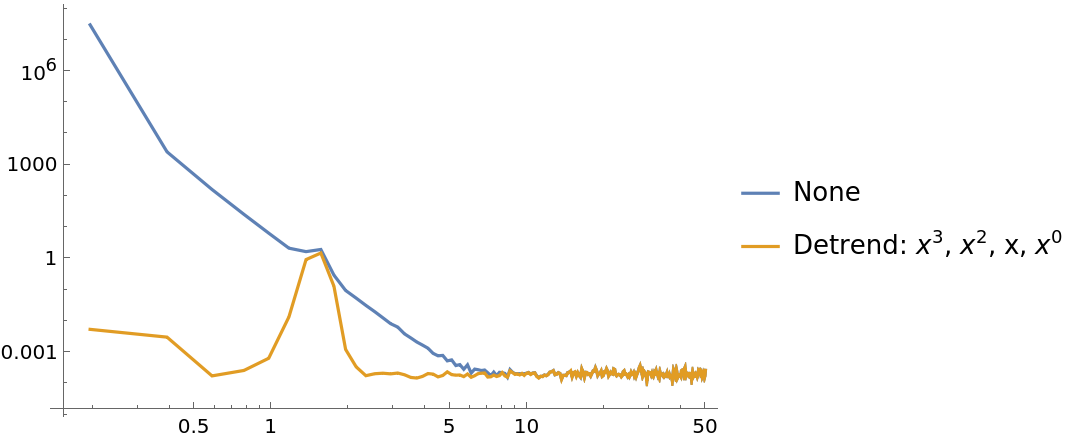
Detrend (2)
The "Detrend" option can be used to detrend the initial data segments before transformations occur. The option can be specified with string arguments for constant or linear detrends:
A list of integers can be specified instead which polynomial orders should be detrended:
Applications (2)
Investigate the frequency response on white noise data for different kind of filters:
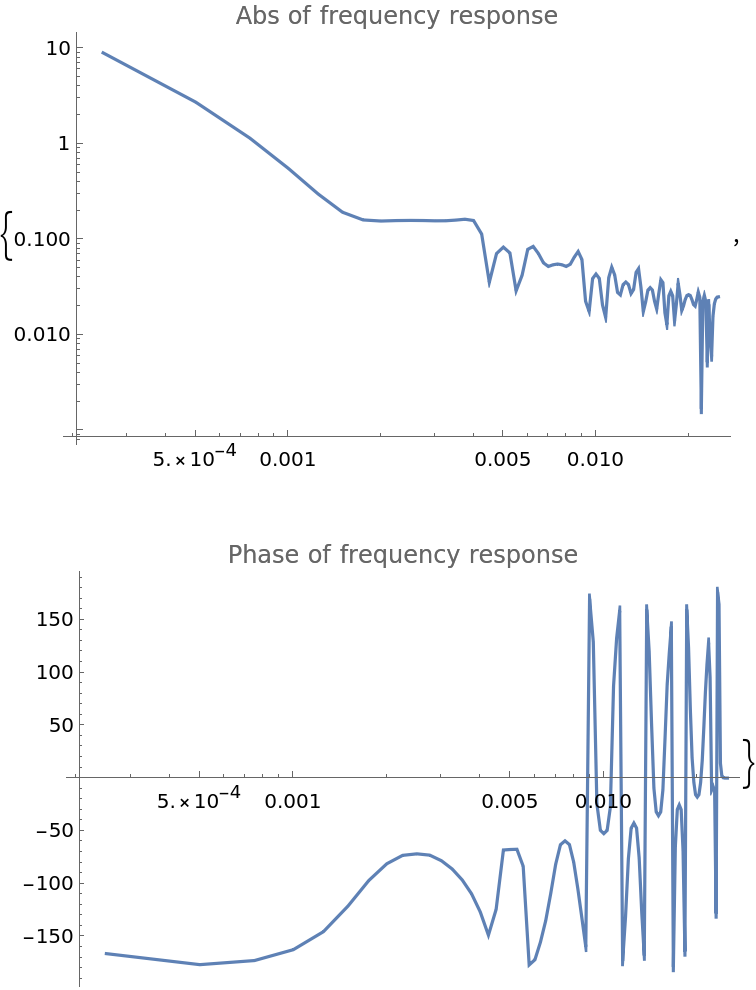
Estimate the system transfer function of a set state and its measured response:
Possible Issues (1)
Even for complex input, the "OneSided" option will still remain True by default and thus return only the front of the Fourier transform:
Neat Examples (1)
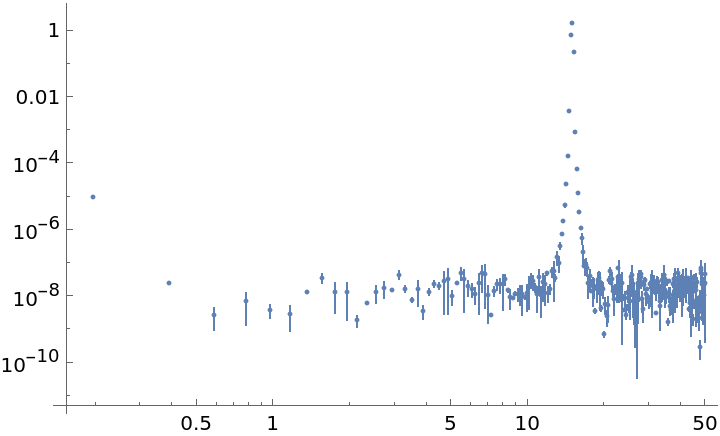
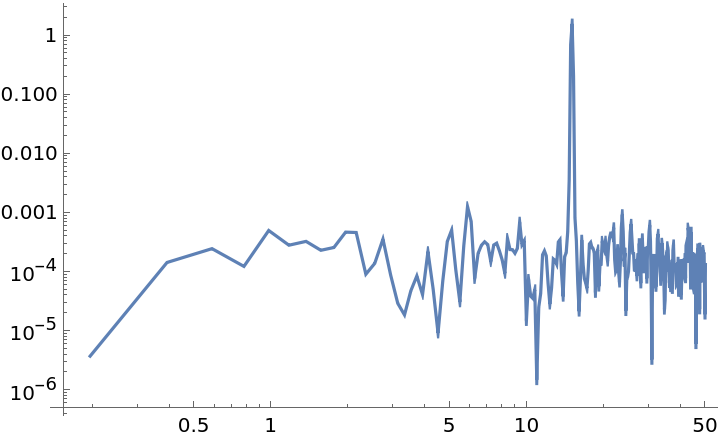
Estimate the uncertainty of the Fourier deviations by just choosing a different reduction function:
Publisher
Julien Kluge
Related Links
Version History
-
1.0.1
– 04 April 2025
-
1.0.0
– 10 February 2021
![data1 = Sin[15*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.1], 1001];
data2 = 0.3 Sin[15*2 Pi*Range[0, 10, 0.01] + 0.5] + Sin[10*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.1], 1001];
csd = ResourceFunction["WelchSpectralEstimate"][data1, data2, 100.0];
{ListLogLogPlot[Abs[csd], Joined -> True], ListLogLinearPlot[{#1, Arg@#2} & @@@ csd, Joined -> True]}](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/7111d36eb627dcc6.png)
![data = Sin[15*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.001], 1001];
windows = {DirichletWindow, HannWindow, HammingWindow, BlackmanHarrisWindow, KaiserWindow};
ListLogLogPlot[
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Window" -> #] & /@ windows, Joined -> True, PlotLegends -> windows]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/7e7466d126d0c6cf.png)
![data = Sin[15*2 Pi*Range[0, 10, 0.001]] + RandomVariate[NormalDistribution[0, 0.1], 10001];
ListLogLogPlot[
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "SegmentSize" -> #], Joined -> True, PlotLabel -> #] & /@ {200, 500, 1000, 5000, 10000}](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/10486ddb6496651e.png)
![data = Sin[15*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.1], 1001];
ListLogLogPlot[
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "OverlapOffset" -> #], Joined -> True, PlotLabel -> #] & /@ {1, 511}](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/07738ca380b5b8cd.png)
![data = Sin[15*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.1], 1001];
ListLogLogPlot[
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "OverlapOffset" -> #], Joined -> True, PlotLabel -> #] & /@ {0.01,
0.99}](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/0d6199b60ca88fa1.png)
![data = Sin[15*2 Pi*Range[0, 10, 0.01]] + RandomVariate[NormalDistribution[0, 0.1], 1001];
ListLogLogPlot[
ResourceFunction["WelchSpectralEstimate"][data, 100.0, FourierParameters -> {1, -1}], Joined -> True]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/1cd59dd4464c7b02.png)
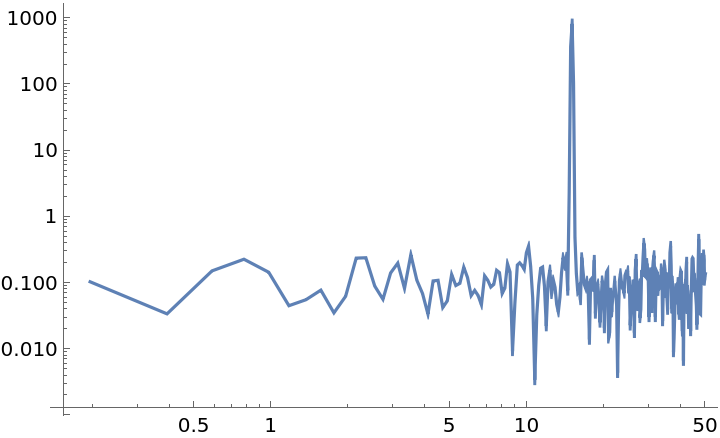
![data = Sin[15*2 Pi*Range[0, 10, 0.001]] + RandomVariate[NormalDistribution[0, 0.1], 10001];
ListLogLogPlot[{
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Window" -> DirichletWindow],
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Window" -> HannWindow]
}, Joined -> True, PlotLegends -> {"Dirichlet", "Hann"}]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/45b34da55fe5bfb8.png)
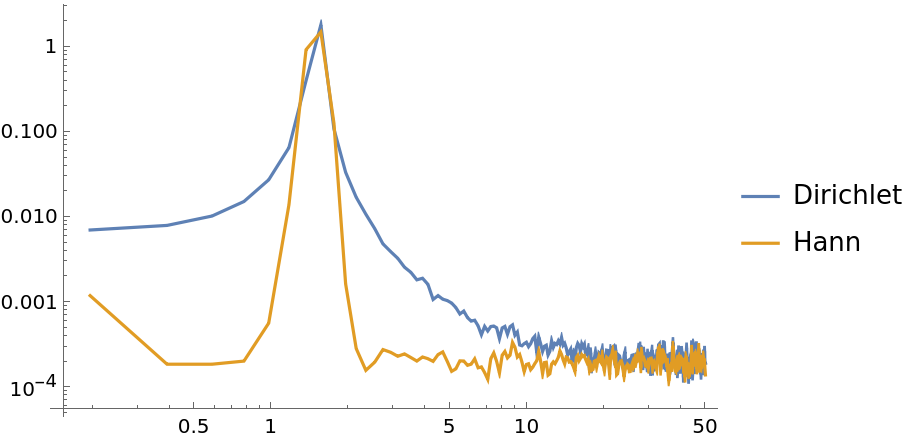
![data = Sin[15*2 Pi*Range[0, 10, 0.001]] + RandomVariate[NormalDistribution[0, 0.1], 10001];
segmentSize = 1024;
windowPoints = PDF[NormalDistribution[0, 0.1], Subdivide[-1, 1, segmentSize - 1]];
ListLogLogPlot[{ResourceFunction["WelchSpectralEstimate"][data, 100.0,
"SegmentSize" -> segmentSize, "Window" -> windowPoints]}, Joined -> True]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/117514f7880d7f4f.png)
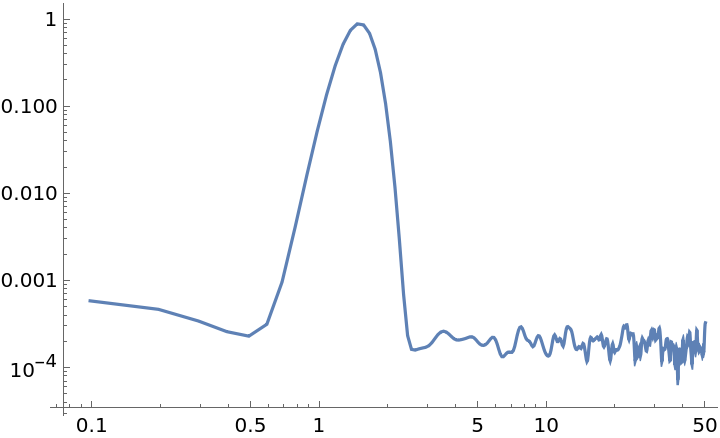
![data = Sin[15*2 Pi*Range[0, 10, 0.001]] + RandomVariate[NormalDistribution[0, 0.1], 10001];
ListLogLogPlot[{
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Window" -> (Exp[-50 #^2] &)],
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Window" -> (HannWindow[#, 0.4] &)]
}, Joined -> True]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/521769c098925449.png)
![(* Evaluate this cell to get the example input *) CloudGet["https://www.wolframcloud.com/obj/08505304-8fb8-4f9a-8650-67d7c32ff920"]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/243d54faa9326b72.png)
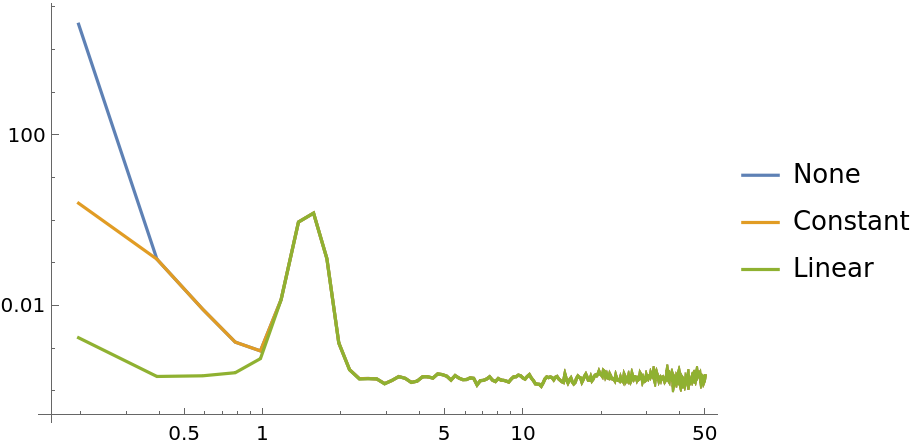
![data = Sin[15*2 Pi*Range[0, 10, 0.001]] + 10*Range[0, 10, 0.001] + 10*Range[0, 10, 0.001]^2 + 10*Range[0, 10, 0.001]^3 + 100 + RandomVariate[NormalDistribution[0, 0.1], 10001];
ListLogLogPlot[{
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Detrend" -> None],
ResourceFunction["WelchSpectralEstimate"][data, 100.0, "Detrend" -> {0, 1, 2, 3}]
}, Joined -> True, PlotLegends -> {"None", "Detrend: \!\(\*SuperscriptBox[\(x\), \(3\)]\), \!\(\*SuperscriptBox[\(x\), \(2\)]\), x, \!\(\*SuperscriptBox[\(x\), \(0\)]\)"}]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/3c5ab89d8ee2a3f6.png)
![(* Evaluate this cell to get the example input *) CloudGet["https://www.wolframcloud.com/obj/07e1b8a6-d841-4a80-99fc-593947cfa61c"]](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/0730c7fcb59be957.png)
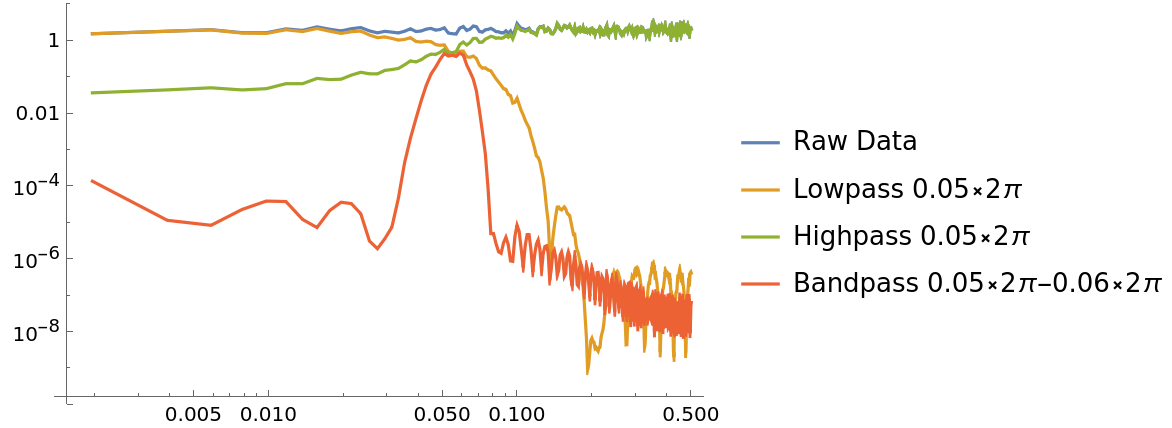
![time = Range[0, 10, 0.05];
data = Table[Round[Mod[x, 1]] - 0.5, {x, time}];
dataResponse = NDSolveValue[{u'[
t] == (Interpolation[Transpose[{time, data}], InterpolationOrder -> 0][t] - u[t]), u[0] == 0}, u, {t, Min[time], Max[time]}] /@ time;
csd = ResourceFunction["WelchSpectralEstimate"][data, dataResponse, 0.05, "Window" -> KaiserWindow];
psd = ResourceFunction["WelchSpectralEstimate"][data, 0.05, "Window" -> KaiserWindow];
transferFunction = Transpose[{csd[[All, 1]], csd[[All, 2]]/psd[[All, 2]]}];
phase = Arg[transferFunction[[All, 2]]];
{
ListLogLogPlot[{#1, Abs@#2} & @@@ transferFunction, Joined -> True, PlotLabel -> "Abs of frequency response", ImageSize -> Medium],
ListLogLinearPlot[{#1, 180/Pi*Arg@#2} & @@@ transferFunction, Joined -> True, PlotRange -> All, PlotLabel -> "Phase of frequency response", ImageSize -> Medium]
}](https://www.wolframcloud.com/obj/resourcesystem/images/bbe/bbee4715-d7fe-426a-a646-e433c01a904d/1-0-0/17af4e7a75d6c5b5.png)