Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Generate the canonical form of a convex polyhedron
ResourceFunction["PolyhedronCanonicalForm"][poly] gives the canonical form of the convex polyhedron poly. |
| MaxIterations | 100 | maximum number of iterations to use |
| Tolerance | Automatic | tolerance to be used for internal comparisons |
| WorkingPrecision | MachinePrecision | the precision used in internal computations |
The tetrahedrally stellated icosahedron:
| In[1]:= |
| In[2]:= |
| Out[2]= | 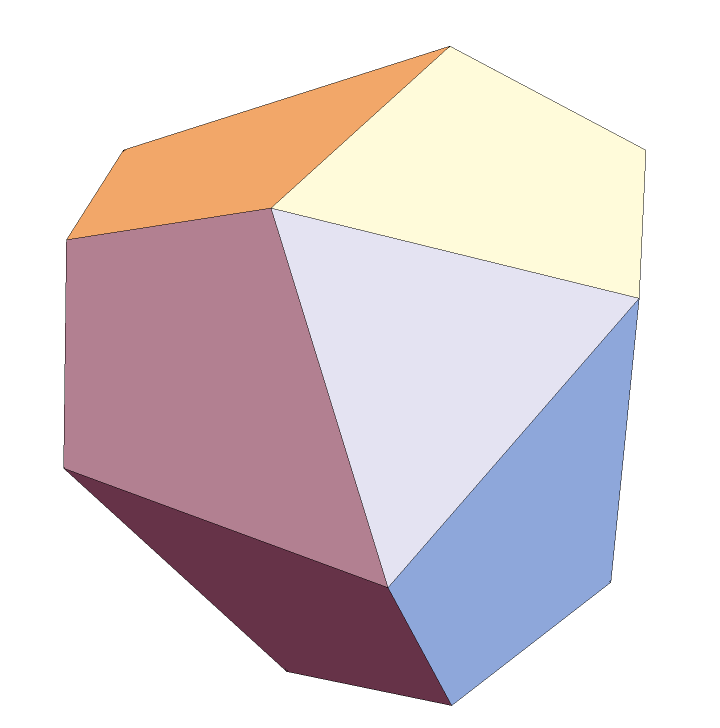 |
The canonical form of the polyhedron:
| In[3]:= |
| Out[3]= |
| In[4]:= |
| Out[4]= | 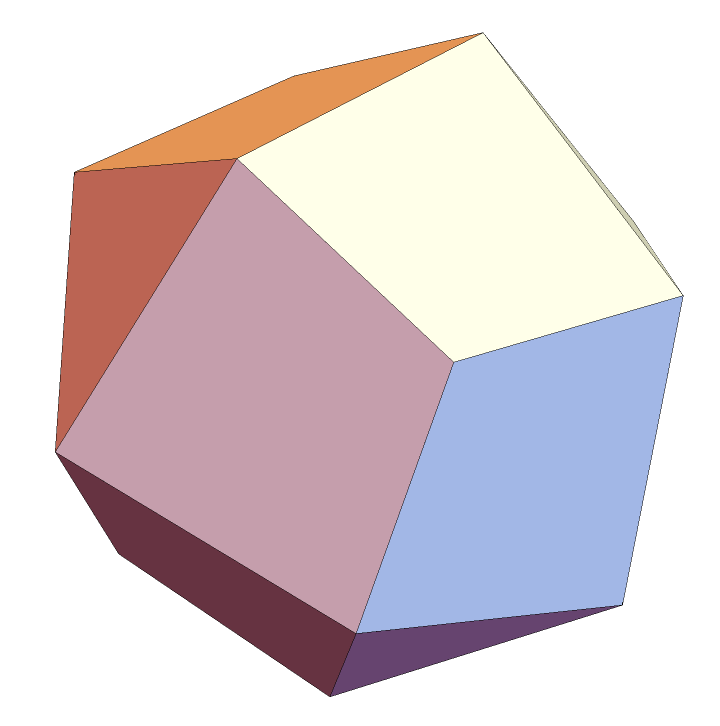 |
PolyhedronCanonicalForm can be used on MeshRegion or BoundaryMeshRegion objects:
| In[5]:= |
| Out[5]= | 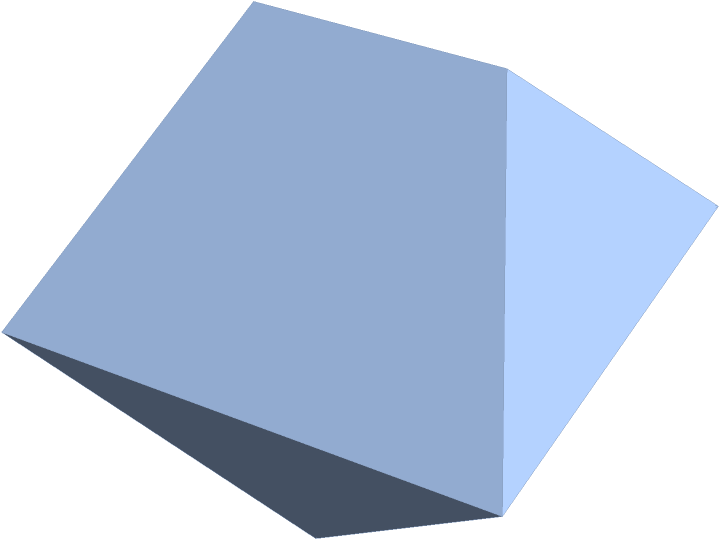 |
Use a smaller number of iterations:
| In[6]:= |
| Out[6]= |
Use a looser tolerance:
| In[7]:= |
| Out[7]= |
Use a higher precision setting in internal computations:
| In[8]:= | ![ResourceFunction[
"PolyhedronCanonicalForm"][Polyhedron[{{-0.5, 0., 0.}, {0., -0.5, 1.5678618484651274`}, {0., 0.5, 1.5678618484651274`}, {
0., -0.644584273224155, 0.5783693583793041}, {0., 0.6445842732241549, 0.5783693583793041}, {0.5, 0., 0.}, {-0.644584273224155, 0., 0.9894924900858232}, {
0.6445842732241549, 0., 0.9894924900858232}}, {{2, 3, 7}, {3, 2, 8}, {4, 2, 7}, {2, 4, 8}, {6, 8, 4}, {4, 1, 6}, {7, 1, 4}, {3, 5, 7}, {8, 5, 3}, {5, 8, 6}, {5, 6, 1}, {5, 1, 7}}], WorkingPrecision -> 25]](https://www.wolframcloud.com/obj/resourcesystem/images/6b4/6b4cfc4f-82fa-4663-bdc5-2706ff15b898/0e852b86b14030df.png) |
| Out[8]= |
Define J. H. Conway's "hermaphrodite" polyhedron:
| In[9]:= | ![conwayHermaphrodite[n_Integer] := Polyhedron[
Join[Table[{Cos[2 \[Pi] i/n], Sin[2 \[Pi] i/n], -1}, {i, n}], Table[{Cos[2 \[Pi] i/n], Sin[2 \[Pi] i/n], 0}, {i, n}], {{0, 0, 1}}], Join[{Range[n], {1, n + 1, 2 n, n}, {n + 1, 2 n, 2 n + 1}},
Table[{i, i + 1, n + i + 1, n + i}, {i, n - 1}], Table[{n + i, n + i + 1, 2 n + 1}, {i, n - 1}]]]](https://www.wolframcloud.com/obj/resourcesystem/images/6b4/6b4cfc4f-82fa-4663-bdc5-2706ff15b898/3dd3faeafef46b32.png) |
| In[10]:= |
| Out[10]= |  |
Visualize the canonical form:
| In[11]:= |
| Out[11]= | 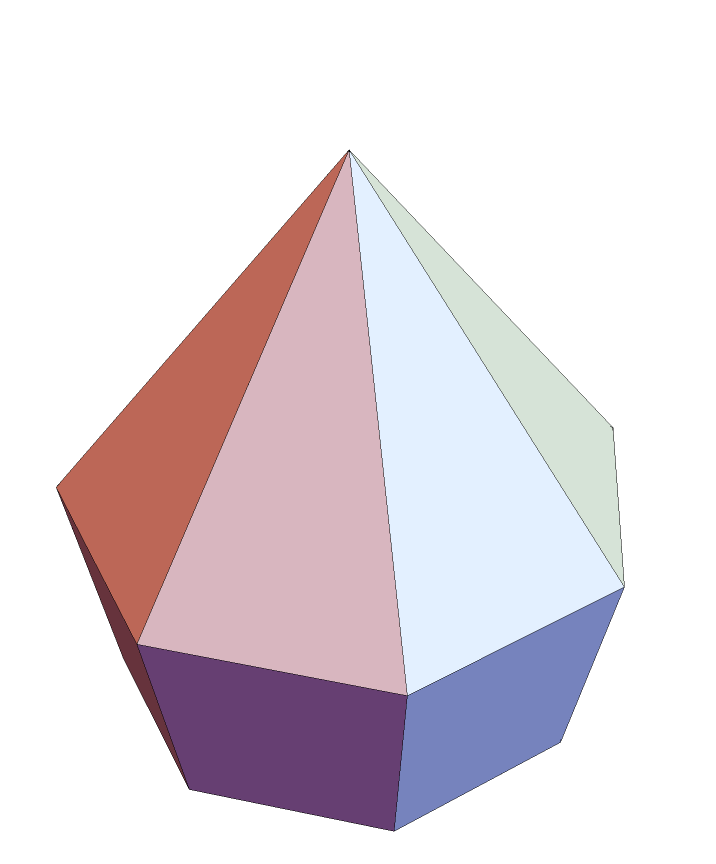 |
The canonical form is self-dual:
| In[12]:= |
| Out[12]= | 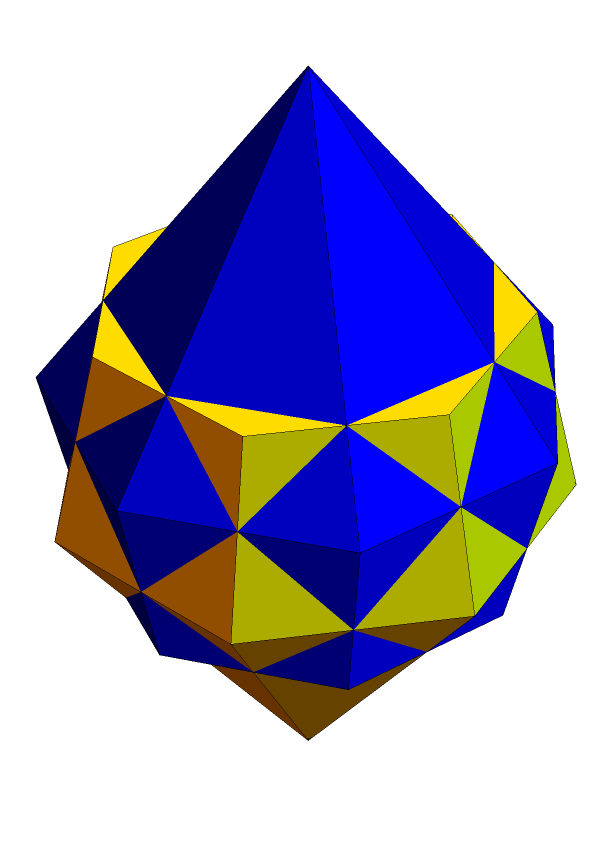 |
This work is licensed under a Creative Commons Attribution 4.0 International License