Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Return a lollipop graph composed of a clique and a path graph
ResourceFunction["LollipopGraph"][n,m] gives a lollipop graph composed of a clique of size n and a undirected path graph of size m. |
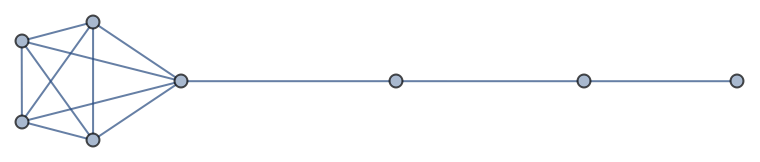
| In[1]:= |
| Out[1]= | 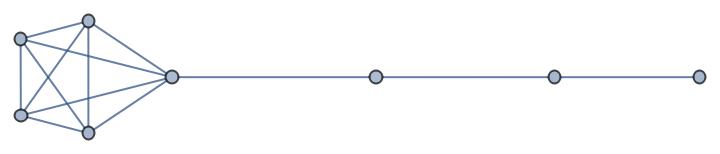 |
Specify an annotation for vertices:
| In[2]:= |
| Out[2]= |  |
Edges:
| In[3]:= |
| Out[3]= |  |
Highlight the vertex 1:
| In[4]:= |
| Out[4]= | 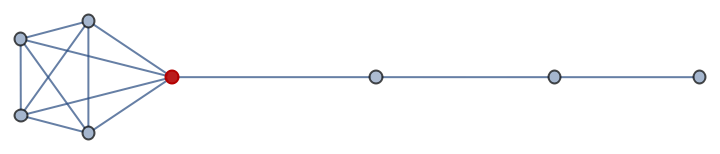 |
Highlight the edge 23:
| In[5]:= |
| Out[5]= | 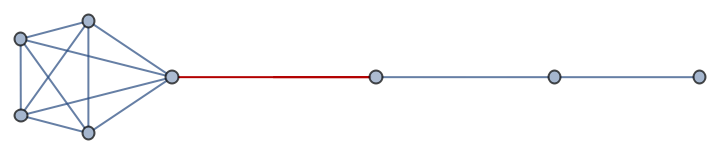 |
Highlight the vertices and edges:
| In[6]:= |
| Out[6]= | 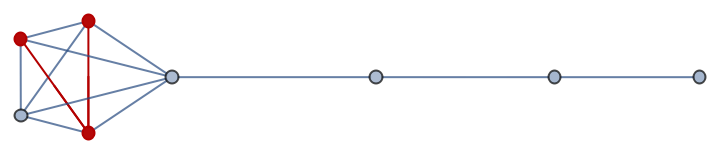 |
Use a common base theme:
| In[7]:= |
| Out[7]= | 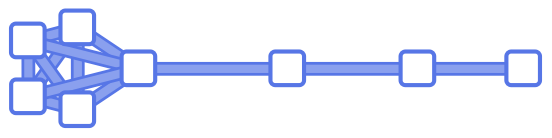 |
Use a monochrome theme:
| In[8]:= |
| Out[8]= |  |
By default, the size of vertices is computed automatically:
| In[9]:= |
| Out[9]= |  |
Specify the size of all vertices using symbolic vertex size:
| In[10]:= | ![Table[ResourceFunction["LollipopGraph"][5, 3, VertexSize -> s, PlotLabel -> s, ImageSize -> Small], {s, {Tiny, Small, Medium, Large}}]](https://www.wolframcloud.com/obj/resourcesystem/images/4de/4debb60c-d3e9-41f4-826f-6adff491f5ed/06d6a508ae7c9bb2.png) |
| Out[10]= | 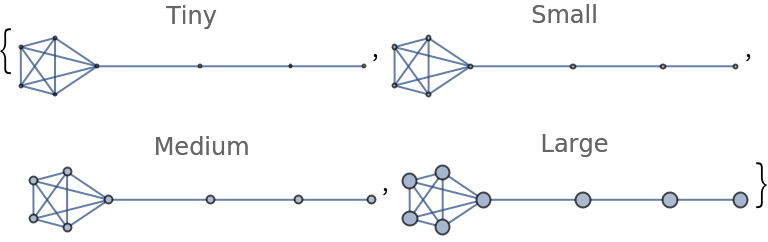 |
Specify the size for individual vertices:
| In[11]:= |
| Out[11]= | 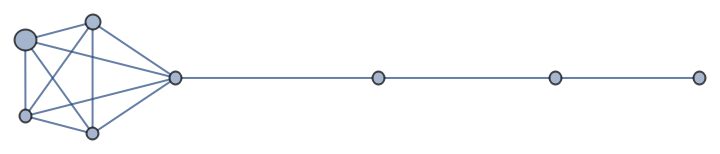 |
Style all vertices:
| In[12]:= | ![Table[ResourceFunction["LollipopGraph"][5, 3, VertexStyle -> style, VertexSize -> 0.3, PlotLabel -> ToString[style], ImageSize -> Small], {style, {Yellow, EdgeForm[Dashed]}}]](https://www.wolframcloud.com/obj/resourcesystem/images/4de/4debb60c-d3e9-41f4-826f-6adff491f5ed/15b399a19f488b48.png) |
| Out[12]= | 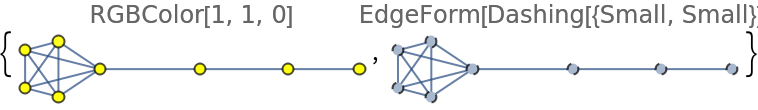 |
Style individual vertices:
| In[13]:= |
| Out[13]= | 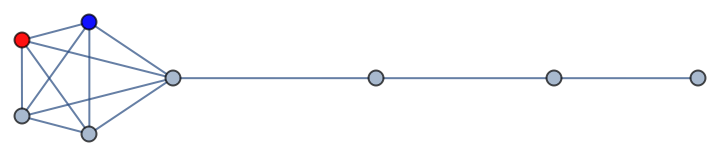 |
Number of vertices of LollipopGraph[{n,m}]is n+m:
| In[14]:= |
| Out[14]= |
| In[15]:= |
| Out[15]= |
Number of edges of LollipopoGraph[{n,m}]is (n(n-1))/2+m:
| In[16]:= |
| Out[16]= |
| In[17]:= |
| Out[17]= |
Wolfram Language 13.0 (December 2021) or above
This work is licensed under a Creative Commons Attribution 4.0 International License