Details
[X1,…,Xn] represents the bracketed product that extends the Lie bracket product called the left-normed bracket, which is defined recursively by
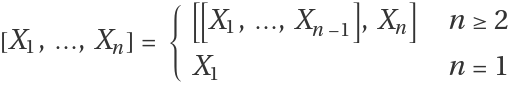
For any Lie algebra, a subset
J of the symmetric group
Sn is said to be a Jacobi subset if it satisfies

.
This identity is also called the
n th-order Jacobi identity of
J.
A Jacobi subset J can be labeled Jk,l for k+l≤n, and Jk,l is called a Jacobi subset of (k,l)-order.
The explicit expression for
Jk,l is represented by the following complex expression.
Despite its complexity, it can be organized using the shuffle
Sh1.
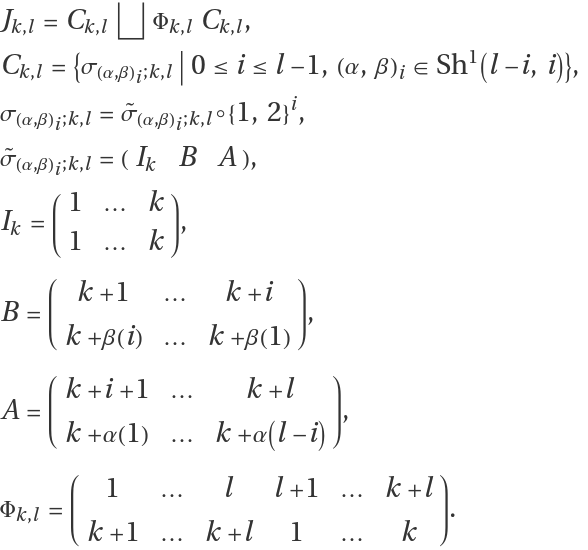
For example, since J1,1={{1,2},{2,1}} for n=2=1+1, the second-order Jacobi identity is the anti-commutator.
And since J1,2=J2,1={{1,2,3},{2,3,1},{3,1,2}} for n=3=1+2=2+1, the third-order Jacobi version is the standard Jacobi identity.
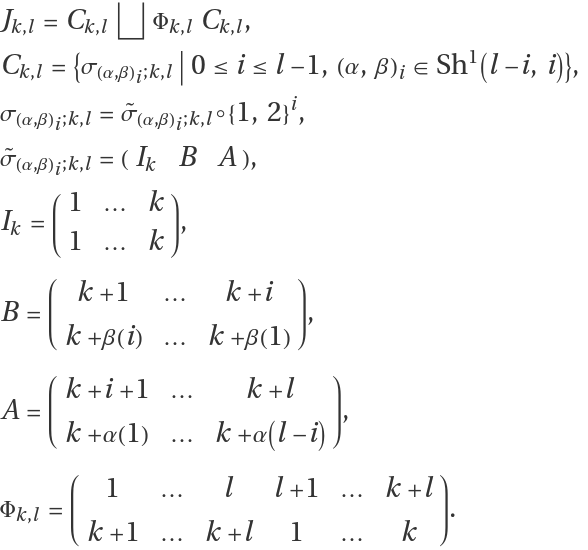
![jacobiator // ClearAll;
jacobiator // Attributes = {};
jacobiator[k_, l_, m_] := Module[{MatrixCommutator},
MatrixCommutator[{mm_}] := mm;
MatrixCommutator[{a_, b_}] := a . b - b . a;
MatrixCommutator[{mm__}] := Fold[MatrixCommutator[{#1, #2}] &][{mm}];
Sum[i, {i, Map[MatrixCommutator[Map[m, #]] &, ResourceFunction["JacobiShufflePermutations"][k, l]]}] // FullSimplify
];
m[k_] := {{Subscript[a, k], Subscript[b, k]}, {Subscript[c, k], Subscript[d, k]}};
{jacobiator[1, 2, m], jacobiator[1, 3, m]}
Clear[jacobiator, m];](https://www.wolframcloud.com/obj/resourcesystem/images/341/34100bb0-73d9-4748-9cd0-99d1aa788819/2661cf7768cca667.png)
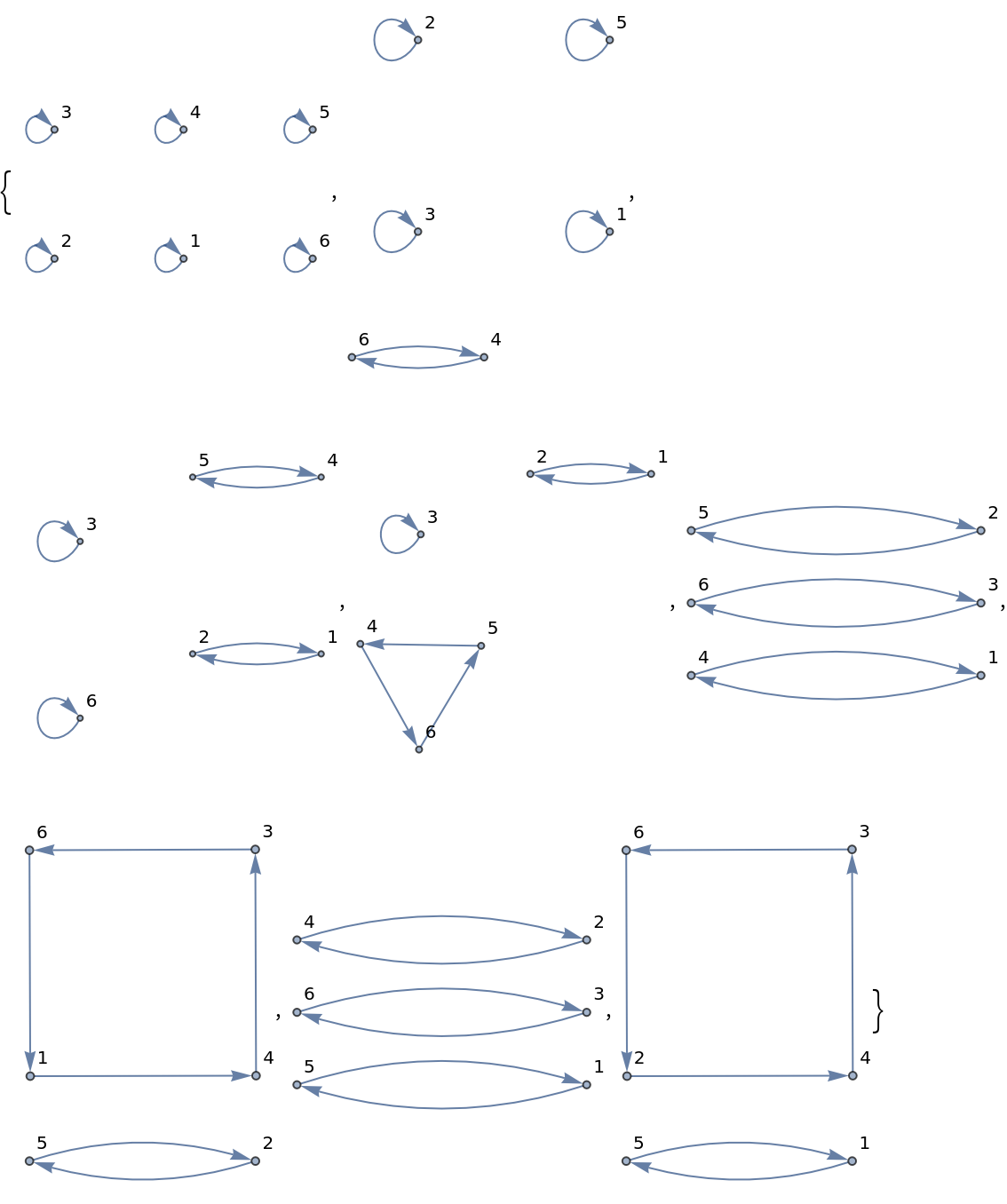
![Table[ResourceFunction["PermutationCyclesGraph"][p, VertexLabels -> "Name"], {p, ResourceFunction["JacobiShufflePermutations"][1, 3]}]](https://www.wolframcloud.com/obj/resourcesystem/images/341/34100bb0-73d9-4748-9cd0-99d1aa788819/4a6035155f35aa1d.png)
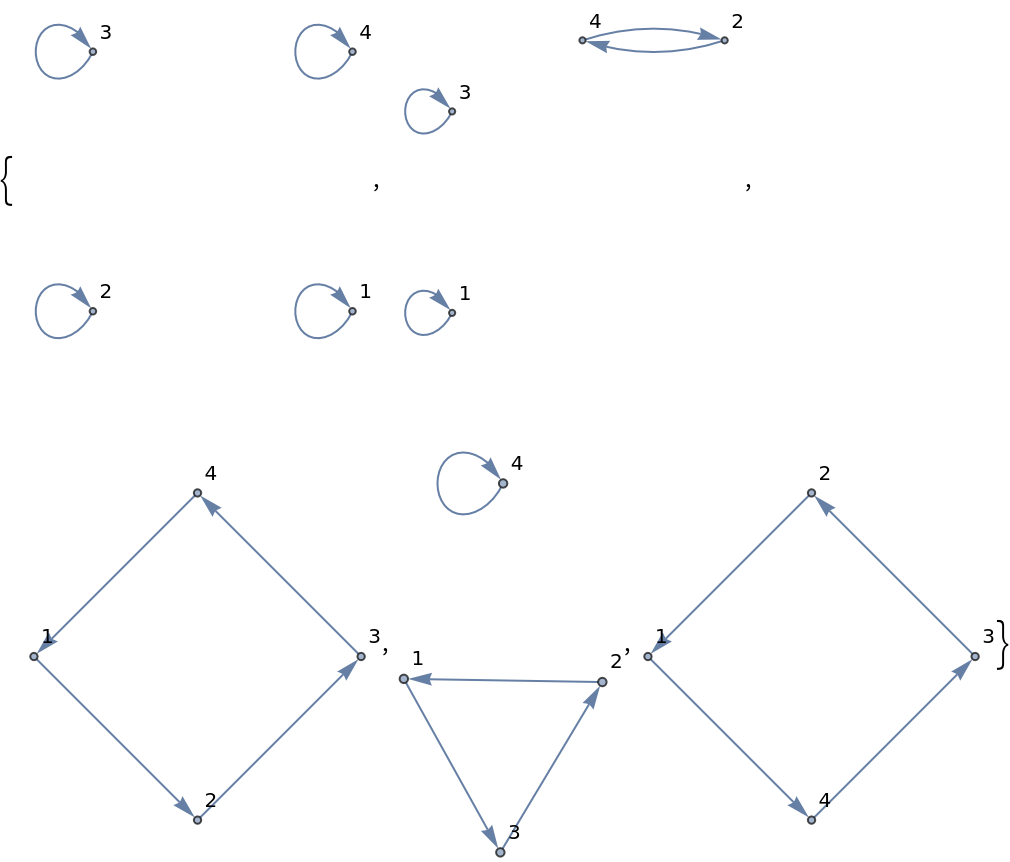
![Table[ResourceFunction["PermutationCyclesGraph"][p, VertexLabels -> "Name"], {p, ResourceFunction["JacobiShufflePermutations"][3, 3]}]](https://www.wolframcloud.com/obj/resourcesystem/images/341/34100bb0-73d9-4748-9cd0-99d1aa788819/7d7c821909e78d9e.png)