Wolfram Function Repository

Instant-use add-on functions for the Wolfram Language

Function Repository Resource:

 FunctionDiscontinuities (10.0.0) current version: 10.0.1 »

Source Notebook

Compute the discontinuities of a function of a single variable

Contributed by: Wolfram|Alpha Math Team

ResourceFunction["FunctionDiscontinuities"][f,x]

computes the values of x at which f(x) is discontinuous with respect to x.

ResourceFunction["FunctionDiscontinuities"][{f,cond},x]

computes the x for which cond is True and f(x) is discontinuous with respect to x.

ResourceFunction["FunctionDiscontinuities"][…,x,"Properties"]

computes points of discontinuity along with related information about each.

Details and Options

ResourceFunction["FunctionDiscontinuities"] has the attribute HoldFirst.
ResourceFunction["FunctionDiscontinuities"] takes the option "ExcludeRemovableSingularities", having default value False, that determines whether to exclude removable discontinuities from the result.
A function f(x) is said to have a removable discontinuity at a point x=a if the limit of f(x) as x→a exists and is independent of the direction in which the limit is taken, but has a value that is different from f(a) (which may or may not be defined).

Examples

Basic Examples (6) 

Compute the points of discontinuity of a rational function:

In[1]:=
ResourceFunction["FunctionDiscontinuities"][(x - 3)/(x + 4), x]
Out[1]=

Repeat the calculation, classifying the points of discontinuity:

In[2]:=
ResourceFunction["FunctionDiscontinuities", ResourceVersion->"10.0.0"][(x - 3)/(x + 4), x, "Properties"]
Out[2]=

Compute the points of discontinuity of a trigonometric function:

In[3]:=
ResourceFunction["FunctionDiscontinuities"][Tan[x], x]
Out[3]=

Repeat the calculation, classifying the points of discontinuity:

In[4]:=
ResourceFunction["FunctionDiscontinuities"][Tan[x], x, "Properties"]
Out[4]=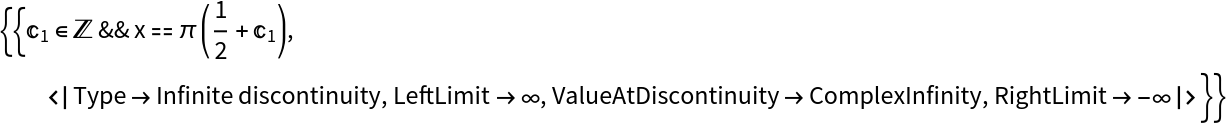

Compute the points of discontinuity of a smooth function:

In[5]:=
ResourceFunction["FunctionDiscontinuities"][E^x *Sin[x], x]
Out[5]=

Compute and plot the points of discontinuity of a rational function:

In[6]:=
f[x_] := (-12 - x + x^2)/(8 + 2 x - 5 x^2 + x^3); ResourceFunction["FunctionDiscontinuities"][f[x], x, "Properties"]
Out[7]=
In[8]:=
Plot[f[x], {x, -5 , 5}, ExclusionsStyle -> {{Red, Dashed}, {PointSize -> 0.02}}]
Out[8]=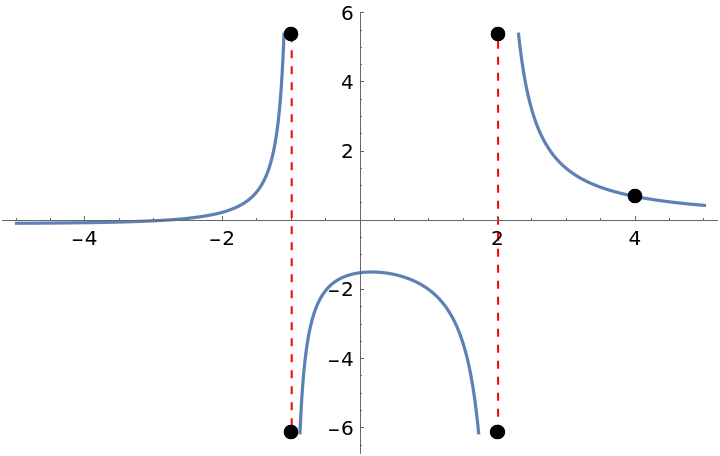

Compute and plot the points of discontinuity of a step function:

In[9]:=
f[x_] := HeavisideTheta[x]; ResourceFunction["FunctionDiscontinuities"][f[x], x, "Properties"]
Out[10]=
In[11]:=
Plot[f[x], {x, -5 , 5}, ExclusionsStyle -> {{Red, Dashed}, {PointSize -> 0.02}}]
Out[11]=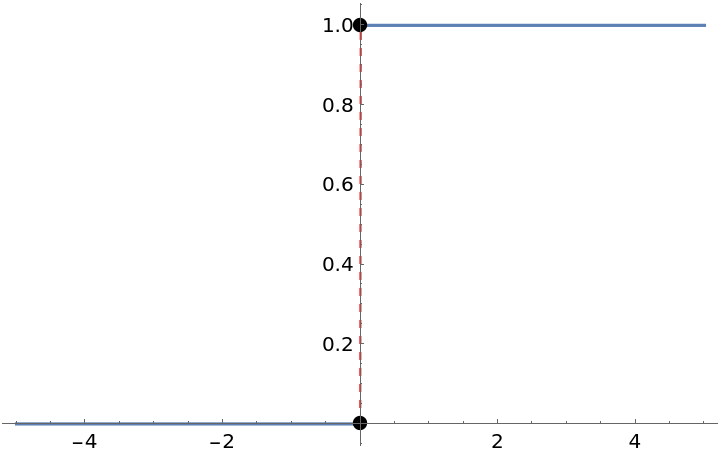

Compute and plot the points of discontinuity of a step function:

In[12]:=
f[x_] := Piecewise[{{Sin[x], x < 1}, {Cos[x], x > 1 && x < 3}}, x - 3]; ResourceFunction["FunctionDiscontinuities"][f[x], x, "Properties"]
Out[13]=
In[14]:=
Plot[f[x], {x, -5 , 5}, ExclusionsStyle -> {{Red, Dashed}, {PointSize -> 0.02}}]
Out[14]=

Options (1) 

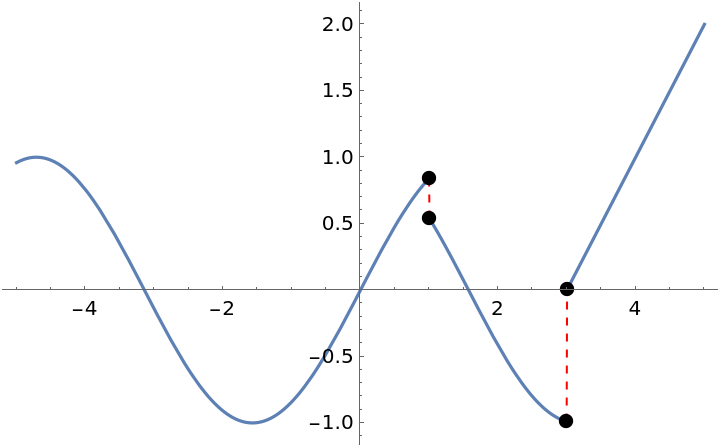Choose whether to exclude removable singularities:

In[15]:=
f[x_] := (x^3 + 8)/(x^3 + 3 x^2 - 4 x - 12); Plot[f[x], {x, -5, 5}, ExclusionsStyle -> {{Red, Dashed}, {PointSize -> 0.02}}]
Out[16]=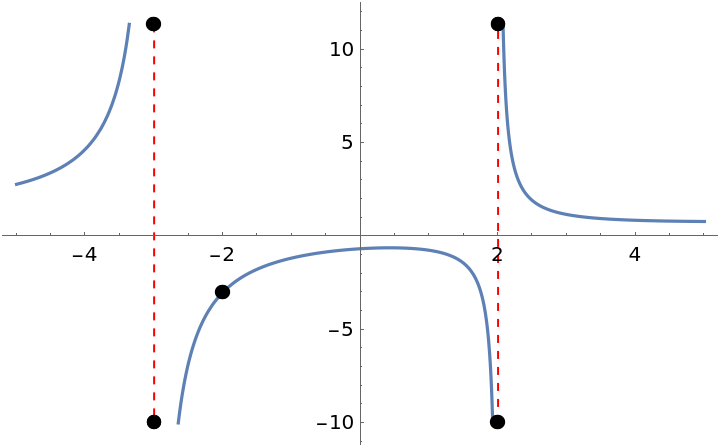
In[17]:=
ResourceFunction["FunctionDiscontinuities"][f[x], x, "ExcludeRemovableSingularities" -> True]
Out[17]=
In[18]:=
ResourceFunction["FunctionDiscontinuities"][f[x], x, "ExcludeRemovableSingularities" -> False]
Out[18]=

Properties and Relations (1) 

FunctionDiscontinuities has the attribute HoldFirst, enabling calculations such as the following:

In[19]:=
ResourceFunction["FunctionDiscontinuities"][x/x, x]
Out[19]=

Possible Issues (1) 

Points where a function approaches ±∞ are considered to be points of discontinuity, even if they are technically outside the range of function definition:

In[20]:=
ResourceFunction["FunctionDiscontinuities"][Log[x], x]
Out[20]=

Publisher

Wolfram|Alpha Math Team

Version History

  • 10.0.1 – 27 March 2023
  • 10.0.0 – 23 March 2023
  • 9.0.0 – 23 March 2023
  • 8.0.0 – 24 March 2020
  • 7.0.0 – 10 September 2019
  • 6.0.0 – 06 September 2019
  • 5.0.0 – 05 September 2019
  • 4.0.0 – 31 July 2019
  • 3.0.0 – 12 June 2019
  • 2.0.0 – 11 June 2019
  • 1.0.0 – 09 January 2019

Related Resources

License Information