Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Get the negabinary representation of an integer
ResourceFunction["ToNegabinary"][n] gives the negabinary representation of the integer n. |
Get the representation for 17 in negabinary:
| In[1]:= |
| Out[1]= |
From this representation, recover the original integer:
| In[2]:= |
| Out[2]= |
Use the resource function FromNegabinary:
| In[3]:= |
| Out[3]= |
Some integers have the same representation in base 2:
| In[4]:= |
| Out[4]= |
ToNegabinary handles negative numbers:
| In[5]:= |
| Out[5]= |
Plot the negabinary representation for the first 22 integers:
| In[6]:= | ![ArrayPlot[
PadLeft[Reverse@
Flatten[Table[
ResourceFunction["ToNegabinary"][n], {n, 85}], {{2}, {1}}], Automatic, None], ColorRules -> {0 -> Gray}, Frame -> False]](https://www.wolframcloud.com/obj/resourcesystem/images/6df/6df8f8c6-075b-4888-a0d8-314b2485d8f1/5dfc246e7b055b8e.png) |
| Out[6]= |
A table of integers and their negabinary representations:
| In[7]:= | ![Grid[Map[Text, Join[{{"n", "(-2\!\(\*SuperscriptBox[\()\), \(4\)]\)=16", "(-2\!\(\*SuperscriptBox[\()\), \(3\)]\)=-8", "(-2\!\(\*SuperscriptBox[\()\), \(2\)]\)=4", "(-2\!\(\*SuperscriptBox[\()\), \(1\)]\)=-2", "negabinary"}}, Table[Flatten[{n, PadLeft[ResourceFunction["ToNegabinary"][n], 4], Row[ResourceFunction["ToNegabinary"][n]]}], {n, -5, 5}]], {2}], Alignment -> Right, Frame -> All]](https://www.wolframcloud.com/obj/resourcesystem/images/6df/6df8f8c6-075b-4888-a0d8-314b2485d8f1/029840d77ac9e988.png) |
| Out[7]= | 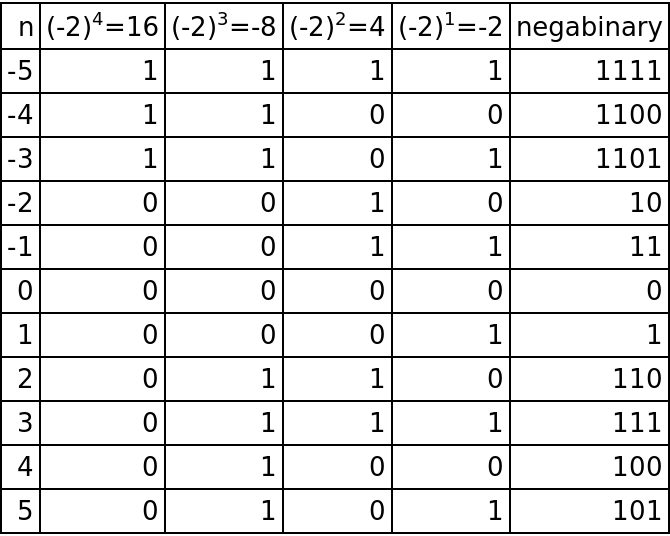 |
Numbers belonging to the Moser–de Bruijn sequence have identical binary and negabinary representations:
| In[8]:= |
| In[9]:= |
| Out[9]= |
| In[10]:= |
| Out[10]= |
Wolfram Language 11.3 (March 2018) or above
This work is licensed under a Creative Commons Attribution 4.0 International License