Wolfram Function Repository
Instant-use add-on functions for the Wolfram Language
Function Repository Resource:
Compute the Galois group for a polynomial
ResourceFunction["GaloisGroupProperties"][poly,var] returns the Galois group for a univariate polynomial poly in the variable var. | |
ResourceFunction["GaloisGroupProperties"][poly,var,prop] returns the specified property prop. |
Compute the Galois group of the polynomial x2+1:
| In[1]:= |
|
| Out[1]= |
|
Compute the Galois group of the polynomial x4+2:
| In[2]:= |
|
| Out[2]= |
|
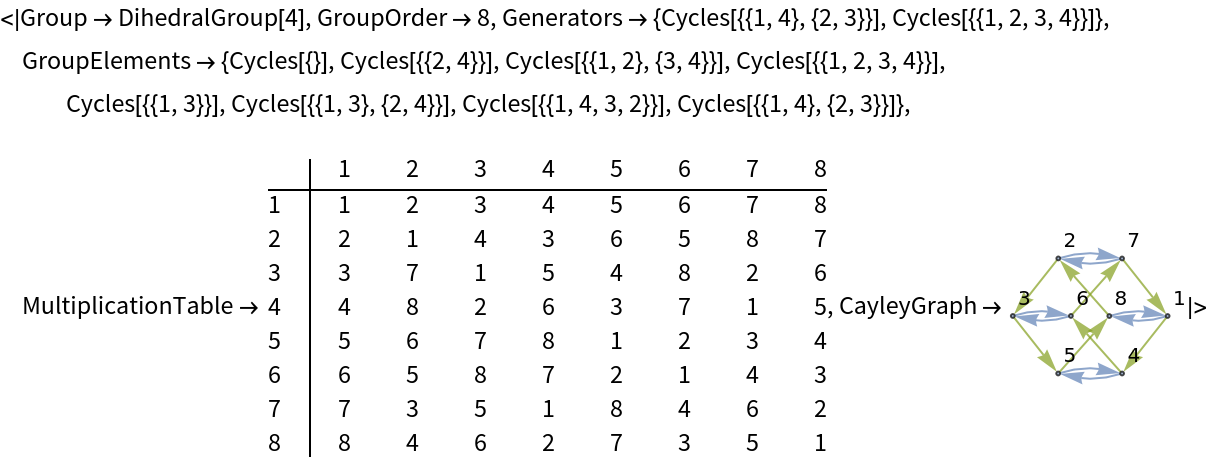
Return the CayleyGraph for the Galois group:
| In[3]:= |
|
| In[4]:= |
![(* Evaluate this cell to get the example input *) CloudGet["https://www.wolframcloud.com/obj/f858d0b7-0bd9-4045-bc75-f9023638a388"]](https://www.wolframcloud.com/obj/resourcesystem/images/053/053539e0-3d79-4077-bfa0-57086b95d370/57e8ef648824604b.png)
|
Find the group order:
| In[5]:= |
|
| Out[5]= |
|
Get the generators:
| In[6]:= |
|
| Out[6]= |
|
Find the group elements:
| In[7]:= |
|
| Out[7]= |
|
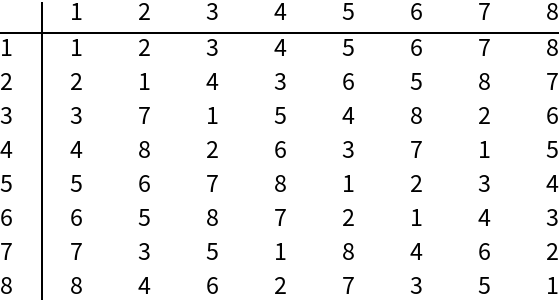
Display the group multiplication table:
| In[8]:= |
|
| Out[9]= |
|
Get all of the available properties as an Association:
| In[10]:= |
|
| Out[10]= |
|
An irreducible polynomial of prime degree p larger than 4 with exactly 2 nonreal roots has Galois group SymmetricGroup[p]:
| In[11]:= |
|
| Out[11]= |
|
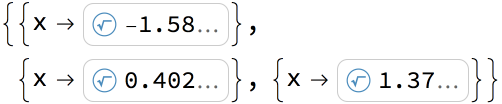
Verify that there are 3 real roots:
| In[12]:= |
|
| Out[12]= |
|
The Galois group for the irreducible polynomial of prime degree 5 with 2 nonreal roots is:
| In[13]:= |
|
| Out[13]= |
|
This work is licensed under a Creative Commons Attribution 4.0 International License